Bài 21 trang 23 Sách giáo khoa (SGK) Hình học 10 Nâng cao

Bài 21. Cho tam giác vuông cân \(OAB\) với \(OA = OB = a\). Hãy dựng các vec tơ sau đây và tính độ dài của chúng

\(\eqalign{
& \overrightarrow {OA} + \overrightarrow {OB} ;\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\;\;\;\overrightarrow {OA} - \overrightarrow {OB} ;\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,3\overrightarrow {OA} + 4\overrightarrow {OB} ; \cr
& {{21} \over 4}\overrightarrow {OA} + 2,5\overrightarrow {OB} ;\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,{{11} \over 4}\overrightarrow {OA} - {3 \over 7}\overrightarrow {OB} . \cr} \)

Hướng dẫn trả lời

Vẽ hình vuông \(OACB\), ta có

\(\eqalign{
& \overrightarrow {OA} + \overrightarrow {OB} = \overrightarrow {OC} \, \Rightarrow \left| {\overrightarrow {OA} + \overrightarrow {OB} } \right| = \left| {\overrightarrow {OC} } \right| = a\sqrt 2 \cr
& \overrightarrow {OA} - \overrightarrow {OB} = \overrightarrow {BA} \, \Rightarrow \left| {\overrightarrow {OA} - \overrightarrow {OB} } \right| = \left| {\overrightarrow {BA} } \right| = a\sqrt 2 \cr} \)

Gọi \(M, N\) là điểm thỏa mãn \(\overrightarrow {OM}  = 3\overrightarrow {OA} ,\,\overrightarrow {ON}  = 4\overrightarrow {OB} \).

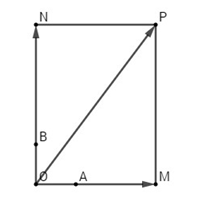
Vẽ hình chữ nhật \(MONP\), ta có

\(\eqalign{
& \overrightarrow {OM} + \overrightarrow {ON} = \overrightarrow {OP} \, \Rightarrow \left| {\overrightarrow {OM} + \overrightarrow {ON} } \right| = \left| {\overrightarrow {OP} } \right| \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \sqrt {O{M^2} + O{N^2}} \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = \sqrt {9{a^2} + 16{a^2}} = 5a \cr
& \cr} \)

Tương tự, ta cũng có

\(\eqalign{
& \left| {{{21} \over 4}\overrightarrow {OA} + 2,5\overrightarrow {OB} } \right| = \sqrt {{{\left( {{{21} \over 4}a} \right)}^2} + {{\left( {{5 \over 2}a} \right)}^2}} = {{\sqrt {541} } \over 4}a\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \cr} \)

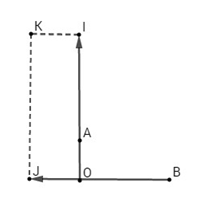
Gọi \(I, J\) là điểm thỏa mãn

 \(\overrightarrow {OI}  = {{11} \over 4}\overrightarrow {OA} ,\,\overrightarrow {OJ}  =  - {3 \over 7}\overrightarrow {OB} \)

Vẽ hình chữ nhật \(OIKJ\), ta có

\(\eqalign{
& {{11} \over 4}\overrightarrow {OA} - {3 \over 7}\overrightarrow {OB} = \overrightarrow {OK} = {{11} \over 4}\overrightarrow {OA} + \left( { - {3 \over 7}\overrightarrow {OB} } \right) = \overrightarrow {OI} + \overrightarrow {OJ} = \overrightarrow {OK} \cr
& \Rightarrow \,\left| {{{11} \over 4}\overrightarrow {OA} - {3 \over 7}\overrightarrow {OB} } \right| = \left| {\overrightarrow {OK} } \right| = \sqrt {{{\left( {{{11} \over 4}a} \right)}^2} + {{\left( { - {3 \over 7}a} \right)}^2}} = {{\sqrt {6073} } \over {28}}a \cr} \)  

Bài 22 trang 23 Sách giáo khoa (SGK) Hình học 10 Nâng cao

Bài 22. Cho tam giác \(OAB\). Gọi \(M, N\) lần lượt là trung điểm hai cạnh \(OA\) và \(OB\). Hãy tìm các số \(m\) và \(n\) thích hợp trong mỗi đẳng thức sau đây

\(\eqalign{
& \overrightarrow {OM} = m\overrightarrow {OA} + n\overrightarrow {OB} ;\,\,\,\,\,\,\overrightarrow {MN} = m\overrightarrow {OA} + n\overrightarrow {OB} ; \cr
& \overrightarrow {AN} = m\overrightarrow {OA} + n\overrightarrow {OB} ;\,\,\,\,\,\,\,\,\overrightarrow {MB} = m\overrightarrow {OA} + n\overrightarrow {OB} . \cr} \)

Hướng dẫn trả lời

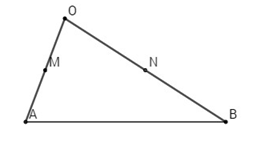
Ta có

\(\eqalign{
& \overrightarrow {OM} = {1 \over 2}\overrightarrow {OA} = {1 \over 2}\overrightarrow {OA} + 0.\overrightarrow {OB} \,\,\,\,\, \Rightarrow \,m = {1 \over 2},\,n = 0. \cr
& \overrightarrow {MN} = \overrightarrow {ON} - \overrightarrow {OM} = {1 \over 2}\overrightarrow {OB} - {1 \over 2}\overrightarrow {OA} = \left( { - {1 \over 2}} \right)\overrightarrow {OA} + {1 \over 2}\overrightarrow {OB} \,\,\,\,\, \Rightarrow \,m = - {1 \over 2},\,n = {1 \over 2}. \cr
& \overrightarrow {AN} = \overrightarrow {ON} - \overrightarrow {OA} = {1 \over 2}\overrightarrow {OB} - \overrightarrow {OA} = \left( { - 1} \right)\overrightarrow {OA} + {1 \over 2}\overrightarrow {OB} \,\,\,\, \Rightarrow \,m = - 1,\,n = {1 \over 2}. \cr
& \overrightarrow {MB} = \overrightarrow {OB} - \overrightarrow {OM} = \overrightarrow {OB} - {1 \over 2}\overrightarrow {OA} = \left( { - {1 \over 2}} \right)\overrightarrow {OA} + \overrightarrow {OB} \,\,\,\, \Rightarrow \,m = - {1 \over 2},\,n = 1. \cr} \)

Bài 23 trang 24 Sách giáo khoa (SGK) Hình học 10 Nâng cao

Bài 30. Gọi \(M\) và \(N\) lần lượt là trung điểm các đoạn thẳng \(AB\) và \(CD\).  Chứng minh rằng

\(2\overrightarrow {MN}  = \overrightarrow {AC}  + \overrightarrow {BD}  = \overrightarrow {AD}  + \overrightarrow {BC} .\) 

Hướng dẫn trả lời

Theo quy tắc ba điểm, ta có

\(\eqalign{
& \overrightarrow {AC} + \overrightarrow {BD} = \left( {\overrightarrow {AM} + \overrightarrow {MN} + \overrightarrow {NC} } \right) + \left( {\overrightarrow {BM} + \overrightarrow {MN} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \left( {\overrightarrow {AM} + \overrightarrow {BM} } \right) + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \overrightarrow 0 + \overrightarrow 0 = 2\overrightarrow {MN} \cr
& \overrightarrow {AD} + \overrightarrow {BC} = \left( {\overrightarrow {AM} + \overrightarrow {MN} + \overrightarrow {ND} } \right) + \left( {\overrightarrow {BM} + \overrightarrow {MN} + \overrightarrow {NC} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \left( {\overrightarrow {AM} + \overrightarrow {BM} } \right) + \left( {\overrightarrow {NC} + \overrightarrow {ND} } \right) \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 2\overrightarrow {MN} + \overrightarrow 0 + \overrightarrow 0 = 2\overrightarrow {MN} \cr} \)

Vậy \(2\overrightarrow {MN}  = \overrightarrow {AC}  + \overrightarrow {BD}  = \overrightarrow {AD}  + \overrightarrow {BC} .\)

Bài 24 trang 24 Sách giáo khoa (SGK) Hình học 10 Nâng cao

Bài 24. Cho tam giác \(ABC\) và điểm \(G\). Chứng minh rằng

a) Nếu \(\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC}  = \overrightarrow 0 \) thì \(G\) là trọng tâm tam giác \(ABC\);

b) Nếu có điểm \(O\) sao cho \(\overrightarrow {OG}  = {1 \over 3}\left( {\overrightarrow {OA}  + \overrightarrow {OB}  + \overrightarrow {OC} } \right)\) thì \(G\) là trọng tâm tam giác \(ABC\).

Hướng dẫn trả lời

a) Gọi \({G_1}\) là trọng tâm tam giác \(ABC\). Từ đó, ta có \(\overrightarrow {{G_1}A}  + \overrightarrow {{G_1}B}  + \overrightarrow {{G_1}C}  = \overrightarrow 0 .\)

Theo giả thiết, \(\overrightarrow {GA}  + \overrightarrow {GB}  + \overrightarrow {GC}  = \overrightarrow 0 \)

\(\eqalign{
& \Rightarrow \,\overrightarrow {G{G_1}} + \overrightarrow {{G_1}A} + \overrightarrow {G{G_1}} + \overrightarrow {{G_1}B} + \overrightarrow {G{G_1}} + \overrightarrow {{G_1}C} = \overrightarrow 0 \cr
& \Rightarrow \,\,3\overrightarrow {G{G_1}} + \left( {\overrightarrow {{G_1}A} + \overrightarrow {{G_1}B} + \overrightarrow {{G_1}C} } \right) = \overrightarrow {0\,} \,\,\,\,\, \Rightarrow \,\,3\overrightarrow {G{G_1}} = \overrightarrow 0 \,\,\,\,\,\,\, \Rightarrow \,\,\overrightarrow {G{G_1}} = \overrightarrow 0 \,\,\,\, \Rightarrow \,G \equiv {G_1} \cr} \)

b) Gọi \( {G_1}\) là trọng tâm tam giác \(ABC\). Từ đó, ta có \(\overrightarrow {{G_1}A}  + \overrightarrow {{G_1}B}  + \overrightarrow {{G_1}C}  = \overrightarrow 0 .\)

\(\eqalign{
& \overrightarrow {OG} = {1 \over 3}\left( {\overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} } \right) \cr
& = {1 \over 3}\left( {3\overrightarrow {O{G_1}} + \overrightarrow {{G_1}A} + \overrightarrow {{G_1}B} + \overrightarrow {{G_1}C} } \right) = \overrightarrow {O{G_1}} \,\,\,\,\, \Rightarrow \,G \equiv {G_1} \cr} \)

Giaibaitap.me