Bài 1 trang 34 SGK Hình học 10 Nâng cao

Bài 1. Cho tam giác \(ABC\) . Hãy xác định các vec tơ

\(\eqalign{
& \overrightarrow {AB} + \overrightarrow {BC} \,\,;\,\,\,\,\,\,\overrightarrow {CB} + \overrightarrow {BA} \,\,;\,\,\,\,\,\overrightarrow {AB} + \overrightarrow {CA} \,\,;\,\,\,\,\overrightarrow {BA} + \overrightarrow {CB} \,\,;\, \cr
& \overrightarrow {BA} + \overrightarrow {CA} \,\,;\,\,\,\,\,\,\,\overrightarrow {CB} - \overrightarrow {CA} \,\,;\,\,\,\,\,\overrightarrow {AB} - \overrightarrow {CB} \,\,;\,\,\,\,\overrightarrow {BC} - \overrightarrow {AB} \,\,.\, \cr} \)                

Hướng dẫn trả lời

Ta có  

\(\eqalign{
& \overrightarrow {AB} + \overrightarrow {BC} \,\, = \overrightarrow {AC} \,\,\,\,\, \cr
& \overrightarrow {CB} + \overrightarrow {BA} \,\, = \overrightarrow {CA} \,\,\,\,\,\,\,\,\,\, \cr
& \overrightarrow {AB} + \overrightarrow {CA} \,\, = \,\overrightarrow {CA} \, + \,\overrightarrow {AB} \, = \,\overrightarrow {CB} \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \cr
& \overrightarrow {BA} + \overrightarrow {CB} \,\, = \overrightarrow {CB} + \overrightarrow {BA} = \overrightarrow {CA} \cr} \)         

\(\overrightarrow {BA}  + \overrightarrow {CA}  = \overrightarrow {BA}  + \overrightarrow {AD}  = \overrightarrow {BD} \) (Với \(D\) thỏa mãn \(\overrightarrow {CA}  = \overrightarrow {AD} \), tức \(D\) là điểm đối xứng với \(C\) qua \(A\)).

\(\eqalign{
& \overrightarrow {CB} - \overrightarrow {CA} = \overrightarrow {AB} \cr
& \overrightarrow {AB} - \overrightarrow {CB} = \overrightarrow {AB} + \overrightarrow {BC} = \overrightarrow {AC} \cr} \)

\(\overrightarrow {BC}  - \overrightarrow {AB}  = \overrightarrow {BC}  + \overrightarrow {BA}  = \overrightarrow {BE} \) (Với \(E\) là điểm sao cho \(BCEA\) là hình bình hành).

Bài 2 trang 34 SGK Hình học 10 Nâng cao

Bài 2. Cho ba điểm \(O, A, B\) không thẳng hàng. Tìm điều kiện cần và đủ để vec tơ \(\overrightarrow {OA}  + \overrightarrow {OB} \) có giá là đường phân giác của góc AOB.

Hướng dẫn trả lời

Gọi \(C\) là điểm sao cho \(AOBC\) là hình bình hành.

Ta có \(\overrightarrow {OA}  + \overrightarrow {OB}  = \overrightarrow {OC} \),

\(OC\) là phân giác của góc \(AOB\) khi và chỉ khi \(AOB\)C là hình thoi.

\( \Leftrightarrow \,\,OA = OB\).

Bài 3 trang 34 SGK Hình học 10 Nâng cao

Bài 3. Gọi \(O\) là tâm của hình bình hành \(ABCD\). Chứng minh rằng với điểm \(M\) bất kì, ta có

 \(\overrightarrow {MO}  = {1 \over 4}(\overrightarrow {MA}  + \overrightarrow {MB}  + \overrightarrow {MC}  + \overrightarrow {MD} ).\)

Hướng dẫn trả lời

Do \(ABCD\) là hình bình hành nên \(O\) là trung điểm của \(AC, BD\).

Suy ra \(\overrightarrow {OA}  + \overrightarrow {OC}  = \overrightarrow 0 \,,\,\overrightarrow {OB}  + \overrightarrow {OD}  = \overrightarrow 0 \,.\) 

Ta có

\(\eqalign{
& \overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} + \overrightarrow {MD} = \overrightarrow {MO} + \overrightarrow {OA} + \overrightarrow {MO} + \overrightarrow {OB} + \overrightarrow {MO} + \overrightarrow {OC} + \overrightarrow {MO} + \overrightarrow {OD} \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, = 4\overrightarrow {MO} + \overrightarrow {OA} + \overrightarrow {OB} + \overrightarrow {OC} + \overrightarrow {OD} = 4\overrightarrow {MO} \cr
& \Rightarrow \,\,\overrightarrow {MO} = {1 \over 4}(\overrightarrow {MA} + \overrightarrow {MB} + \overrightarrow {MC} + \overrightarrow {MD} ). \cr} \)

Bài 4 trang 34 SGK Hình học 10 Nâng cao

Bài 4. Cho tam giác \(ABC\).

a) Tìm các điểm \(M\) và \(N\) sao cho

\(\overrightarrow {MA}  - \overrightarrow {MB}  + \overrightarrow {MC}  = \overrightarrow 0 \) và \(2\overrightarrow {NA}  + \overrightarrow {NB}  + \overrightarrow {NC}  = \overrightarrow 0 .\)

b) Với các điểm \(M, N\) ở câu a) , tìm các số \(p\) và \(q\) sao cho

\(\overrightarrow {MN}  = p\overrightarrow {AB}  + q\overrightarrow {AC} .\) 

Hướng dẫn trả lời

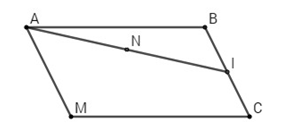
a)  Ta có \(\overrightarrow {MA}  - \overrightarrow {MB}  + \overrightarrow {MC}  = \overrightarrow 0 \, \Leftrightarrow \,\overrightarrow {BA}  + \overrightarrow {MC}  = \overrightarrow 0 \)

\( \Leftrightarrow \,\overrightarrow {CM}  = \overrightarrow {BA} \,.\) Do đó \(ABCM\) là hình bình hành.

Gọi \(I\) là trung điểm của \(BC\), ta có \(\overrightarrow {NB}  + \overrightarrow {NC}  = 2\overrightarrow {NI} \) suy ra \(2\overrightarrow {NA}  + 2\overrightarrow {NI}  = \overrightarrow 0 \)

\( \Rightarrow \,\,\overrightarrow {NA}  + \overrightarrow {NI}  = \overrightarrow 0 \,\,\,\, \Rightarrow \,N\,\) là trung điểm của \(AI\).

b) Từ câu a), ta biểu diễn \(\overrightarrow {AM} ,\,\overrightarrow {AN} \) qua \(\overrightarrow {AB} ,\,\overrightarrow {AC} \).

\(\eqalign{
& \overrightarrow {MA} - \overrightarrow {MB} + \overrightarrow {MC} = \overrightarrow 0 \,\, \Leftrightarrow \, - \overrightarrow {AM} - (\overrightarrow {AB} - \overrightarrow {AM} ) + (\overrightarrow {AC} - \overrightarrow {AM} )=\overrightarrow 0 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \Leftrightarrow \,\overrightarrow {AM} = - \overrightarrow {AB} + \overrightarrow {AC} \cr
& 2\overrightarrow {NA} + \overrightarrow {NB} + \overrightarrow {NC} = \overrightarrow 0 \,\, \Leftrightarrow \, - 2\overrightarrow {AN} + \overrightarrow {AB} - \overrightarrow {AN} + \overrightarrow {AC} - \overrightarrow {AN} = \overrightarrow 0 \cr
& \,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\, \Leftrightarrow \,4\overrightarrow {AN} = \overrightarrow {AB} + \overrightarrow {AC}\Leftrightarrow  \overrightarrow {AN}= {1 \over 4}(\overrightarrow {AB} + \overrightarrow {AC} ) \cr
& \Rightarrow \,\,\overrightarrow {MN} = \overrightarrow {AN} - \overrightarrow {AM} = {1 \over 4}(\overrightarrow {AB} + \overrightarrow {AC} ) + \overrightarrow {AB} - \overrightarrow {AC} = {5 \over 4}\overrightarrow {AB} - {3 \over 4}\overrightarrow {AC} \cr} \)

Vậy \(p = {5 \over 4}\,;\,q =  - {3 \over 4}.\)

Giaibaitap.me